Repetition, Rhythm and Pattern

Motifs

Motifs can be thought of as units of pattern. In visual arts, they are bounded areas or volumes that contain designs or any desired combination of art elements: stamps, tiles, building blocks, modules, etc. Motifs can be copied and arranged in multiple instances to create a desired effect, such as repetition, rhythm and pattern.

Repetition

Repeated use of a shape, color, or other art element or design in a work can help unify different parts into a whole. The repetition might be limited to only an instance or two: not enough to create a pattern or rhythm, but enough to cause a visual echo and reinforce or accent certain aspects of the work.

Rhythm

When motifs or elements are repeated, alternated, or otherwise arranged, the intervals between them or how they overlap can create rhythm and a sense of movement. In visual rhythm, design motifs become the beats. Rhythms can be broadly categorized as random, regular, alternating, flowing, and progressive.

• Random Rhythm - Groupings of similar motifs or elements that repeat with no regularity create a random rhythm. Pebble beaches, the fall of snow, fields of clover, herds of cattle, and traffic jams all demonstrate random rhythms. What may seem random at one scale, however, may exhibit purpose and order at another scale.

  

  René Magritte - Golconde, 1953, oil on canvas, 81 x 100 cm

  
  

  Tom Turner - Oval Bowl Lipped Bottle Vase, 2011, porcelain with oilspot glazes, 8.125 x 5.125 in.

  
  
  

  Chuck Close - Self Portrait 2007 Screenprint, 2007, Screenprint in 187 colors, 74.5 x 57.8 in.




• Regular Rhythm - Like a heart or song with a steady beat, regular rhythm is created by a series of elements, often identical or similar, that are placed at regular or similar intervals, such as in grids. Simple regular rhythms, if overused, can be monotonous.

  

  Jasper Johns - Three Flags, 1958, encaustic on canvas, 30 7/8 × 45 1/2 × 5 in. The flag stripes have alternating rhythm, but the stars and flags themselves have regular rhythm.




• Alternating Rhythm - Two or more different motifs may be alternated, such as the black and red squares in a checkerboard; a single motif might be flipped, mirrored or rotated every so many iterations; or the placement or spacing between motifs can be alternated. This is essentially a regular rhythm that has more complex motifs, or meta-motifs. The added variety can help lessen the monotony of a regular rhythm.

  

  M.C. Escher - Lizard, 1942




• Flowing Rhythm - Flowing rhythm is created by undulating elements and intervals, bending and curving motifs and spaces. Natural flowing rhythm can be seen in streams and waterways, beaches and waves, sand dunes and glaciers, rolling hills and wind-blown grasses.

  

  Gloria Petyarre - Bush Medicine Dreaming, 2008, acrylic on canvas, 152 x 91 cm

  
  

  Steven Hill - Melon Pitcher, 2010, 10.5 x 9 x 7.5 in.




• Progressive Rhythm - In progressive rhythm, each time a motif repeats it changes a little, transforming and translating in a steady sequence - the motif progresses from one thing to another.

  

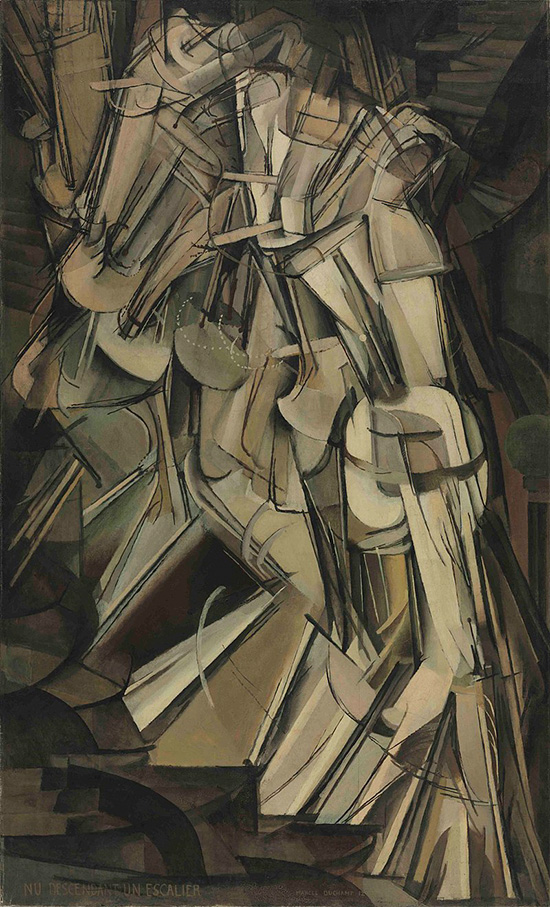
  Marcel Duchamp - Nude Descending a Staircase (No. 2), 1912, oil on canvas, 147 x 89.2 cm

Pattern

Patterns are groups of elements or motifs that repeat in a predictable manner. Artlex lists ten different classes of pattern, given below. I'm not sure where they pulled this out of, but it's enough to get you thinking.

• Spheres

  

  Wayne Mah - ball bearings, 2005

  
  

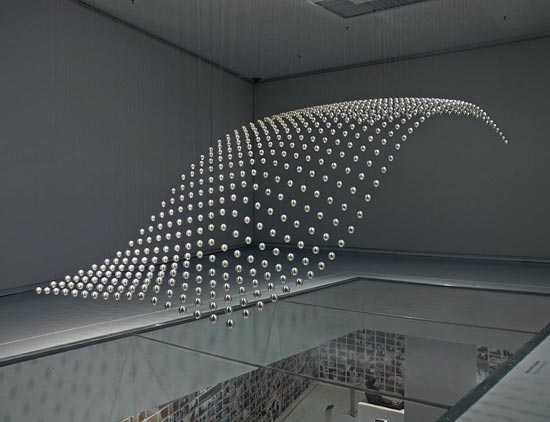
  ART+COM - kinetic sculpture, 2008

  My first guess as to what is meant by spheres being called a class of pattern is how spheres fit or pack together in two and three dimensions. But hey - spheres: they're cool, and you can do a zillion things on their surface or by arranging them in different ways.

• Mosaics or Nests

  Mosaics create patterns from tesserae, small pebbles or cut pieces of stone or glass (traditionally) in different colors. Similar to pixels, these dots or units of color are arranged to create areas of color that form a desired image. Tesserae can be laid in strict grids, but often their arrangement follows the contours of the edges of the color areas they make up. The lines between tesserae, and small areas of a composition, may form random patterns, but viewed from a distance, their arrangement unites to form an image.

  

  Detail, The Empress Theodora and Retinue, The Basilica of St. Vitale, dedicated in 547 A.D.

  
  

  Zac Freeman - Garrett, 2009, Assemblage on board, 26.25 x 33 in.

• Lattices

  Lattices have various definitions in math, science and art. In the main, they can be said to be based around the idea of a two or three-dimensional array of regularly spaced points. Lattices can be classified by the shapes formed between their points: in math these are square, rectangular, parallelgrammatic, rhombic, or hexagonal. Crystal structures and their arrangement of atoms or molecules form lattices in science; in art and architecture, one form of lattices are (or resemble) screens of thin woven or carved materials that usually display a regular structure.

  

  Siddi Sayyed Jali

  
  

  Jali, Humayun's Tomb, Delhi

• Polyhedra

  Polyhedra are three-dimensional objects whose surfaces are defined by polygonal faces or facets, whose edges are in turn defined by straight line segments. These straight line edges meet at points called vertices; each edge joins only two faces and two vertices. Many polyhedra are very symmetrical, but symmetry is not necessary.

  

  Fra Giovanni da Verona - Intarsia Panel From The Church Of Santa Maria In Organo, Verona, circa 1520

  
  

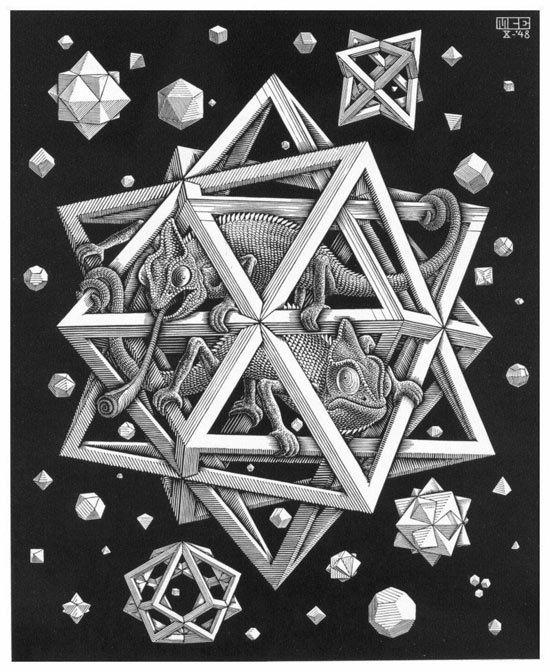
  M.C. Escher - Stars, 1948, wood engraving

  
  

  Jon Barlow Hudson - Double Helix: Flowing Balance, 2008, stainless steel, 9 x 9 x 22 ft.

• Spirals - Helices and Volutes

  • Volutes

    Volutes are a fancy name for flat spirals - what you doodle on your class notes, a slice of nautilus shell, wind-up springs, fiddlehead ferns, our galaxy, etc.

    

    Entrance Stone, Newgrange, County Meath, Ireland - photo by Laurie Young

    
    

    Terracotta jar with three handles - Minoan, 1600-1500 B.C., 13.5 in.

    
    

    Gustav Klimt - The Tree Of Life, 1909, mural

  • Helices

    Helices and double-helices are three-dimensional spirals - think screws, coil springs, DNA, some types of sea/snail shells, and of course, the ever-popular spiral staircase.

    

    Olafur Eliasson - Umschreibung, 2004, steel, 9m high. Photo by Philipp Klinger

    
    

    terrain:loenhart&mayr - Observation Tower on the River Mur, 2020, steel and aluminum, 27m high

    
    

    Andy Goldsworthy, 1997

• Meanders

  Meanders are the sinuous bends that streams and rivers sometimes make, which lend the name to anything with a snaking, winding, convoluted path. Meanders can be thought of as irregular waveforms, as opposed to regular sine waves.

  

  NASA/USGS - Meandering Wadis, Southeastern Jordan, 2001

  
  

  Andy Goldsworthy - Cow dung on glass, 2007

  p.s. - Andy Goldswotrhy is the king of meanders.

• Branching and Circulation

  

  Natalie Blake - Fiddlehead, large, porcelain, 15 x 5.5 in.

  
  

  Kris Pixton - Large Green Plate

  
  

  Sabine+Jones - Ars Electronica exhibition, 2009

  
  

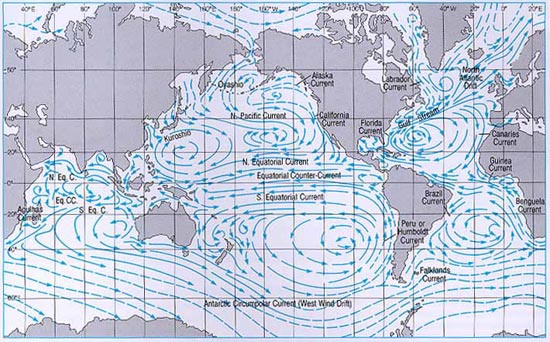
  Ocean Surface Currents Map

• Waves

  Ocean waves, sine waves, sound waves, ripples, etc., and all the designs they inspire.

  

  Akira Satake - kohiki teapot, 8.5 x 5 x 4.5 in.

• Symmetry

  M.C. Escher is the King of Symmetry.

  If you've lived your artistic life happily with bilateral and radial symmetry and symmetric, asymmetric and radial balance, you may wish to skip to the pretty pretty fractals below and live happily ever after. If you find patterns and Escher's work fascinating and want to investigate more, read on. I am going to run screaming through symmetry and tiling, and point you to some more info, or I will be working on this for the rest of my life. Like M.C. Escher.

  Tessellation and Tiling - Tessellation and tiling are created when motifs - units of pattern contained within the boundary of some shape - repeat on a plane without any gaps between the motifs (tiles), and also without overlapping. Common ceramic tiles are a perfect example.

  Symmetries of Planar Patterns - Imagine standing in front of a wall covered with patterned wallpaper, and you have an extra sample of the wallpaper in your hand. You can place your sample against the wall and line it up so it perfectly fits in with the rest of the pattern. Once you have done that, there are four possible ways you can again move (transform) the sample to get it to fit in with the rest of the pattern. These ways depend upon what type of symmetry the pattern has. The four types of planar symmetries, or isometries (transformations that preserve distance) are translation, reflection, rotation, and glide reflection symmetry. In other words, depending upon the pattern's isometry, you might be only able to slide (translate) the wallpaper sample over in a couple directions to get it to fit in again. Perhaps rotating the sample would also cause it to line up with the rest of the wall. If the pattern were printed on both sides of the sample and you could flip it over in some direction (like a mirror's reflection) and get it to fit, the pattern would have reflective isometry. Glide reflection isometry involves flipping the sample along an axis and then sliding it along the axis into place.

  It turns out that if you classify planar patterns by all their isometries, you come up with 17 different plane pattern symmetry groups. And if you really like to break things down, you can further subdivide these into 51 periodic patterns.

  
  

  M.C. Escher - Bird Fish, 1938. This pattern has only translational symmetry. It can be slid along two axis, which is the minimum for the pattern to fill a plane. (If the pattern only slid in one direction, it would form a strip.)

  
  

  M.C. Escher - Study of Regular Division of the Plane With Angels and Devils. This pattern has both reflective and rotational symmetry.

  
  

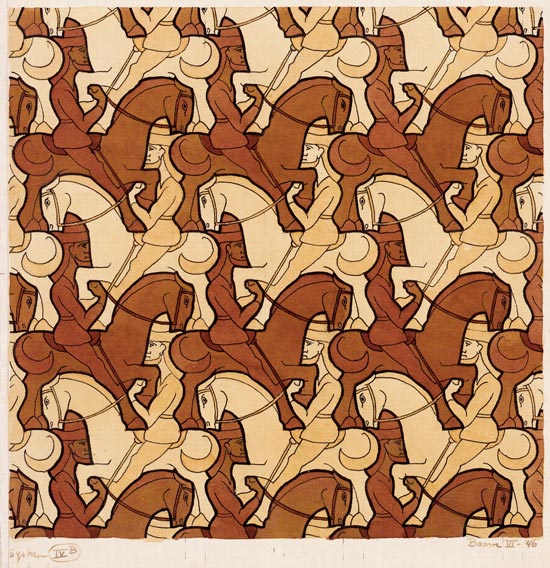
  M.C. Escher - Horseman, 1946. This pattern demonstrates glide reflection. There is no pure reflectional symmetry or rotational symmetry.

  
  

  Further Reading and Examples:

  • Art and Science - by Linda Pearce of Cal State Northridge. Check out her Math in Art page.
  • Wallpaper Groups by David E. Joyce, Clark University - includes good examples of plane symmetry groups.
  • Wallpaper Groups on Wikepedia
  • M.C. Escher's Symmetry Drawings - click on "Picture Gallery", then "Symmetry; most of M.C. Escher's Symmetry Drawings".
  • Tessellations.org

• Fractals

  Fractals are shapes or forms that divide into smaller-scale copies of the themselves, so that they appear similar at any level of magnification. Snow flakes, river systems, and cauliflower are some things that approximate fractals in nature.

  

  Mandelbrot set

  
  

  Mandelbrot set

  
  

  Jock Cooper - Limited Palette Fractal